The Mathematics Behind Snowflakes

During winter, we can find inspiration to investigate some of the most fundamental aspects of the world falling from the sky; simple snowflakes. Their symmetries can lead us to the mathematics of so-called group theory that helps scientists all over the world as well as at the Institute of Science and Technology (IST) Austria to uncover new discoveries in mathematics, physics, and many other fields.

Snowflakes are not only beautiful to look at, they can also teach us something about the underlying mathematical structure in our world: group theory. It’s essential in many fields of abstract and applied mathematics and even leads to major implications in fundamental physics, chemistry, or computer science.

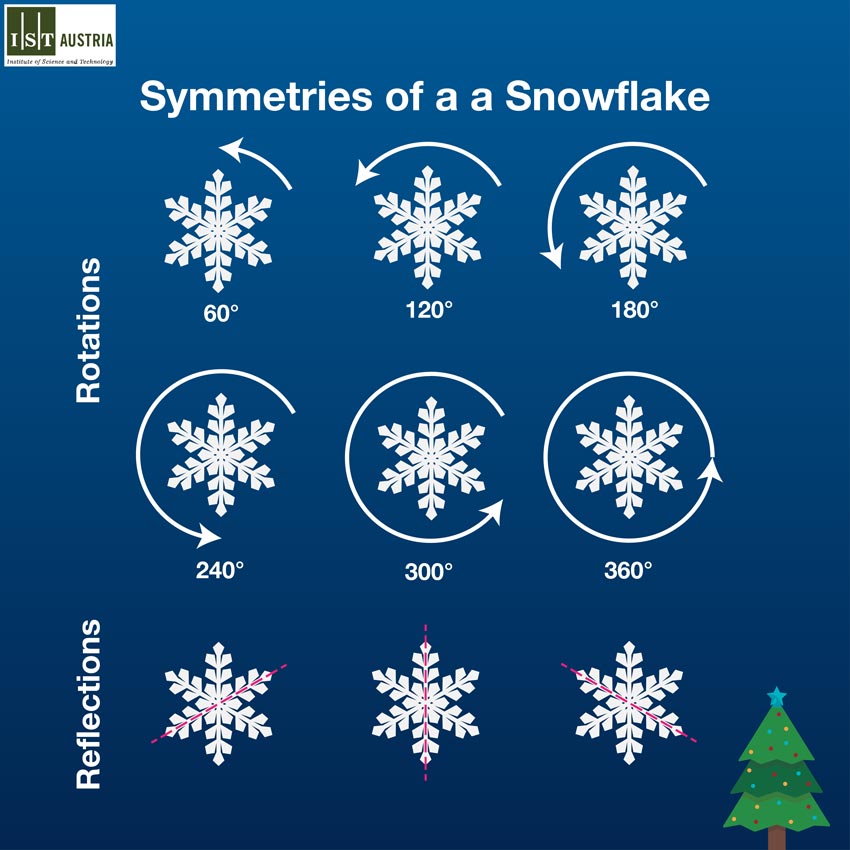
At the Institute of Science and Technology (IST) Austria, several researchers are working with group theory to investigate open questions in mathematics. One of them is Adam Brown, a postdoc researcher in the Edelsbrunner group. He is fascinated by this field’s ability to connect disparate parts of science using symmetry. “Symmetry helps us uncover hidden connections between very different subjects. Some of the most exciting achievements in mathematics combine techniques from different disciplines, such as number theory and geometry, by illuminating their shared patterns and symmetries.”

Playing with Snow

However, what does this have to do with snowflakes? A typical snowflake has a beautiful six-sided symmetry and this is from where our path to group theory begins. In mathematics, a symmetry just means that something does not change its appearance and characteristics under certain transformations. As illustrated in the image above, an admittedly idealized snowflake looks just the same after certain rotations or flips around certain axes. The important thing is to understand that the result of a combination of any two such transformations could itself be described by another transformation. In the picture below, you can see how the combination of two transformations has the same result as just one other transformation. This is the secret of group theory.

In mathematics, a group is a set of things with very specific characteristics. In the case of a snowflake, its inherent symmetries lead to a set of transformations with certain rules of how they can be combined to form new transformations. These rules are what define a group.

Interestingly, a closely related group also arises from the symmetries of a line of three points that can switch places or from studying the solutions of cubic polynomial equations. Here, we can see the otherwise hidden connections between different fields in mathematics. This is a crucial point about group theory: Its somewhat generic name hints at the fact that it lies behind a lot of mathematical structures in our world.

Buckyballs, Algorithms, and Particle Physics

The applications of group theory range widely. For example, understanding the symmetries of carbon-based molecules using group theory helped in the discovery of the so-called Buckyball molecule. It even earned the scientists working on it a Nobel Prize in 1996. “I find it amazing that this deep notion of a symmetry group was used to identify a molecule,” Tamás Hausel, mathematician and professor at IST Austria adds. He and his colleagues are studying much more abstract aspects of groups connecting disparate fields of mathematics.

In computer science, group theory is part of the mathematical toolbox used to study algorithm. It can tell scientists about the complexity of a computation and help them to optimize programs by exploiting the given problem’s symmetries. In physics, group theory plays an important role in quantum mechanics and particle physics telling researchers about the fundamental rules of the universe. The symmetries of the particles’ characteristics dictate how they interact. However, scientists are puzzled by the great mystery that some particles seem to not follow the rules imposed by the symmetries – these particles break the symmetry. Furthermore, even the laws of conservation of energy and momentum are deeply rooted in the symmetries of our universe that can be studied with group theory. It goes to show that a simple thing like a snowflake can lead us to delve deeply into the mathematical structure of our world.